The Ultimate Mathematical Introduction Lecture Notes: Your Path to Mathematical Mastery in 2023!

Are you fascinated by the beauty and precision of mathematics? Do you want to deepen your understanding of this timeless subject? Look no further than the Mathematical Lecture Notes in Mathematics 2023. In this comprehensive guide, we will explore the intricacies of mathematics, providing you with a solid foundation to excel in your mathematical journey. Get ready to embark on a mind-blowing adventure through the world of numbers, formulas, and proofs!

Why Should You Care About Mathematics?

Mathematics is the language of our universe. From understanding the patterns in nature to solving complex real-life problems, mathematics provides us with the tools to make sense of the world around us. Whether you're pursuing a career in science, engineering, finance, or any other field, a strong foundation in mathematics is essential for success. Mathematical skills are highly valued and sought after, as they enable you to think critically, analyze data, and make informed decisions.

Introducing the Mathematical Lecture Notes in Mathematics 2023

The Mathematical Lecture Notes in Mathematics 2023 is a comprehensive collection of lecture notes that cover a wide range of fundamental mathematical topics. These lecture notes have been meticulously crafted by renowned mathematicians and educators, with the aim of providing students and enthusiasts alike with a comprehensive and accessible resource.

Damped Oscillations of Linear Systems: A Mathematical Introduction (Lecture Notes in Mathematics Book 2023)

by Krešimir Veselić(1st Edition, Kindle Edition)

4.6 out of 5

Language	:	English
File size	:	3664 KB
Print length	:	215 pages
Screen Reader	:	Supported
X-Ray for textbooks	:	Enabled

FREE

The lecture notes are divided into various chapters, each focusing on a specific topic. From basic arithmetic and algebra to calculus, differential equations, and linear algebra, you will find everything you need to build a solid mathematical foundation. The notes are structured in a manner that allows for easy navigation and understanding, ensuring a smooth learning experience.

Key Features of the Lecture Notes

1. Comprehensive Coverage: The lecture notes cover a wide range of mathematical topics, making them suitable for beginners as well as those seeking to deepen their mathematical knowledge.

2. Clarity and Accessibility: The notes are written in a clear and concise manner, making complex concepts easy to understand. The use of examples, diagrams, and step-by-step explanations ensures that you grasp the material effortlessly.

3. In-depth Explanations: The lecture notes go beyond rote memorization and delve into the underlying principles of mathematics. You will gain a deeper understanding of the why behind mathematical concepts, enabling you to apply them in various contexts.

4. Practice Problems and Solutions: To solidify your understanding, the lecture notes include a plethora of practice problems with detailed solutions. These problems allow you to apply what you've learned and reinforce your skills through practice.

5. Visual Representations: Visual aids such as graphs, diagrams, and illustrations are incorporated throughout the lecture notes, enhancing your comprehension and making abstract concepts more tangible.

Why Choose the Mathematical Lecture Notes in Mathematics 2023?

1. Learn at Your Own Pace: The lecture notes provide you with the flexibility to learn at your own pace. Whether you prefer studying late at night or during the day, you can access the notes anytime and anywhere, allowing for a customized learning experience.

2. Affordable and Accessible: Unlike expensive textbooks, the Mathematical Lecture Notes in Mathematics 2023 are affordable and easily accessible. They are available in both digital and print formats, catering to your personal preferences.

3. Suitable for Beginners and Advanced Learners: Whether you're a novice or an experienced mathematician looking to brush up on your skills, these lecture notes are designed to accommodate learners of all levels. The comprehensive nature of the notes ensures that you won't miss out on any fundamental concepts, regardless of your prior knowledge.

4. Expertly Crafted by Renowned Mathematicians: The lecture notes are authored by distinguished mathematicians who have extensive experience in teaching and research. You can trust that the content is accurate, reliable, and communicated in a manner that facilitates effective learning.

The Journey to Mathematical Mastery Starts Now!

The Mathematical Lecture Notes in Mathematics 2023 are your ultimate guide to mastering the intricacies of mathematics. With their comprehensive coverage, clear explanations, and extensive practice problems, you will build a strong foundation and gain the confidence to tackle even the most challenging mathematical concepts.

Embrace your passion for mathematics, unlock the secrets of this captivating subject, and embark on a journey that will expand your mind and open doors to countless opportunities. Don't miss out on the Mathematical Lecture Notes in Mathematics 2023 – your gateway to mathematical mastery!

Damped Oscillations of Linear Systems: A Mathematical Introduction (Lecture Notes in Mathematics Book 2023)

by Krešimir Veselić(1st Edition, Kindle Edition)

4.6 out of 5

Language	:	English
File size	:	3664 KB
Print length	:	215 pages
Screen Reader	:	Supported
X-Ray for textbooks	:	Enabled

FREE

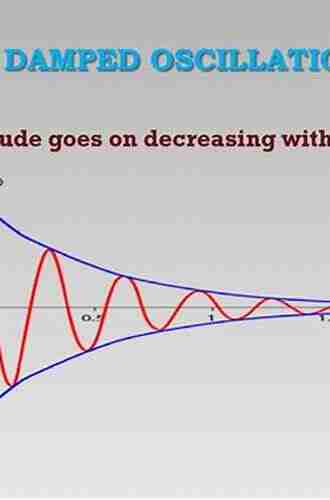
The theory of linear damped oscillations was originally developed more than hundred years ago and is still of vital research interest to engineers, mathematicians and physicists alike. This theory plays a central role in explaining the stability of mechanical structures in civil engineering, but it also has applications in other fields such as electrical network systems and quantum mechanics.
This volume gives an to linear finite dimensional damped systems as they are viewed by an applied mathematician. After a short overview of the physical principles leading to the linear system model, a largely self-contained mathematical theory for this model is presented. This includes the geometry of the underlying indefinite metric space, spectral theory of J-symmetric matrices and the associated quadratic eigenvalue problem. Particular attention is paid to the sensitivity issues which influence numerical computations. Finally, several recent research developments are included, e.g. Lyapunov stability and the perturbation of the time evolution.