Unraveling the Fascinating History of Set Theory and Its Profound Impact on Modern Mathematics

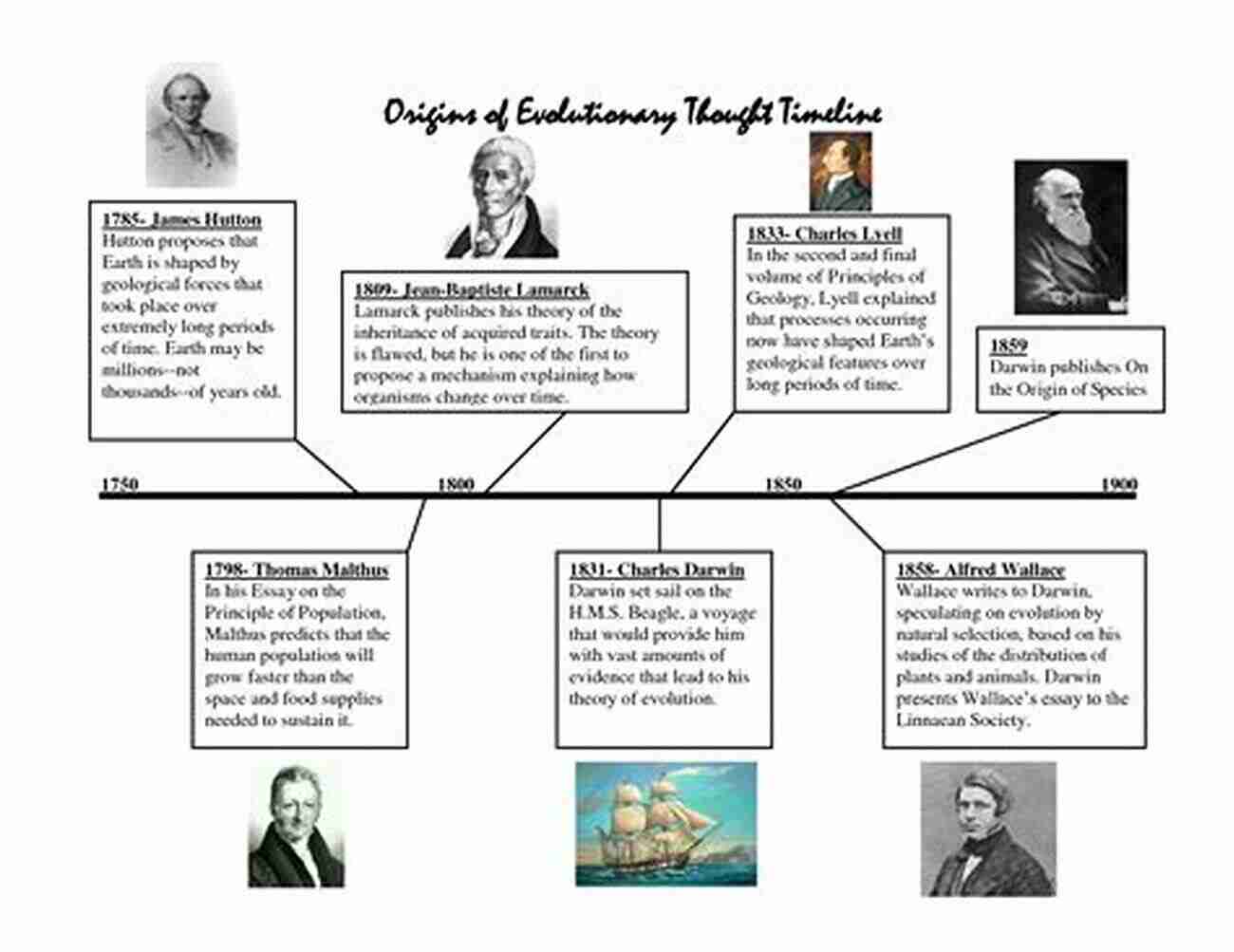
Set theory, a branch of mathematical logic, has had a profound and lasting impact on the development of modern mathematics. From its humble origins in the late 19th century to its far-reaching implications in various mathematical disciplines, its study and theories have reshaped our understanding of mathematical concepts and paved the way for groundbreaking discoveries.

The Birth of Set Theory

The origins of set theory can be traced back to the work of German mathematician Georg Cantor in the late 19th century. Cantor introduced the concept of a "set" as a collection of distinct objects with well-defined properties. His groundbreaking contributions laid the foundation for set theory, revolutionizing the study of mathematical infinites and infinite sets.

One of Cantor's most significant achievements was the discovery of different sizes of infinities. He demonstrated that not all infinities are equal by employing a technique known as "diagonalization." This revolutionary notion challenged the traditional belief that all infinities were essentially the same.

Labyrinth of Thought: A History of Set Theory and Its Role in Modern Mathematics

by José Ferreirós(2nd Edition, Kindle Edition)

5 out of 5

Language	:	English
File size	:	38893 KB
Screen Reader	:	Supported
Print length	:	492 pages
X-Ray for textbooks	:	Enabled

FREE

Set theory, as developed by Cantor, provided a rigorous and systematic framework for analyzing the nature of infinite collections, resolved age-old paradoxes, and opened up entirely new realms of mathematical exploration.

Formalizing Set Theory: Zermelo-Fraenkel Axioms

In the early 20th century, mathematicians Ernst Zermelo and Abraham Fraenkel sought to establish a formal foundation for set theory. Building upon Cantor's work, they formulated a set of axioms known as the Zermelo-Fraenkel axioms (ZF axioms).

These axioms provided a solid logical framework for discussing sets and addressing various foundational questions in mathematics. ZF axioms include axioms of extensionality, pairing, union, power set, and infinity, among others. These axioms, along with the axiom of choice, form ZFC set theory, widely accepted as the standard foundation of contemporary mathematics.

The Impact of Set Theory on Mathematical Disciplines

The development of set theory brought about a paradigm shift in several mathematical disciplines, leading to groundbreaking advancements. Let's explore a few areas where set theory plays a crucial role.

Topology and Analysis

Set theory serves as a fundamental tool in the field of topology, which studies the properties of space that are preserved under continuous deformations. The notion of a set is essential in defining open sets, closed sets, and other fundamental concepts in topology. The ability to manipulate sets and their properties allows for the formulation of powerful theorems and the exploration of various topological spaces.

In analysis, the mathematical study of limits, continuity, and calculus, set theory plays an indispensable role. The concepts of sets, subsets, and functions provide a solid foundation for the study and analysis of real numbers, sequences, and series. Set theory enables the rigorous formulation of limit theorems, continuity principles, and key topics in calculus.

Logic and Foundations of Mathematics

Set theory also has a significant impact on the field of mathematical logic and the foundations of mathematics. The study of formal logic and proofs heavily relies on set theory as a tool for representing mathematical objects and their relationships. By employing sets, logical symbols, and axioms, mathematicians can construct precise and unambiguous mathematical arguments.

Furthermore, set theory plays a crucial role in addressing foundational questions in mathematics. The study of the foundations of mathematics seeks to derive the basic principles and axioms upon which the entire mathematical framework rests. Set theory, with its well-defined axioms and logical framework, provides a solid foundation for constructing and analyzing mathematical theories.

The Future Prospects of Set Theory

As modern mathematics continues to evolve, set theory remains a critical component for further exploration and discovery. The ongoing study of large cardinals, infinity, and the inner structure of sets continues to captivate the imagination of mathematicians.

Set theory's influence is not limited to mathematics alone. It also finds applications in areas such as computer science and theoretical physics. Set theory provides the basis for understanding data structures, algorithm analysis, and the theoretical underpinnings of computation. Additionally, set-theoretic methods contribute to the formulation of physical theories by elucidating foundational issues and quantifying infinite structures.

The history of set theory is a mesmerizing journey through the evolution of mathematical thought. From Cantor's groundbreaking work to the formalization of set theory by Zermelo and Fraenkel, this field has transformed the way mathematicians understand infinity, space, and logical foundations.

Set theory continues to shape modern mathematics, permeating various disciplines and serving as a powerful tool for mathematical analysis, logic, and the foundations of mathematics. With its ongoing developments, set theory remains poised to unravel new mysteries and advance our understanding of the mathematical universe.

Labyrinth of Thought: A History of Set Theory and Its Role in Modern Mathematics

by José Ferreirós(2nd Edition, Kindle Edition)

5 out of 5

Language	:	English
File size	:	38893 KB
Screen Reader	:	Supported
Print length	:	492 pages
X-Ray for textbooks	:	Enabled

FREE

"José Ferreirós has written a magisterial account of the history of set theory which is panoramic, balanced, and engaging. Not only does this book synthesize much previous work and provide fresh insights and points of view, but it also features a major innovation, a full-fledged treatment of the emergence of the set-theoretic approach in mathematics from the early nineteenth century." --Bulletin of Symbolic Logic (Review of first edition)